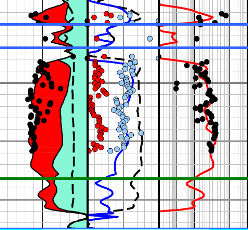
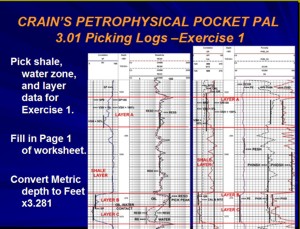
|
Your complete online Petrophysics Reference Manual
covering Petroleum, Minerals, and other Essential Commodities for
the Energy Transition
Loading Table of Contents...
|
||
|
Page Views ---- Since 01 Jan 2015
Copyright 2024 by Accessible Petrophysics Ltd. CPH Logo, "CPH", "CPH Gold Member", "CPH Platinum Member", "Crain's Rules", "Meta/Log", "Computer-Ready-Math", "Petro/Fusion Scripts" are Trademarks of the Author |
||


|
||
| Site Navigation | CHAPTER and TOPIC INDEX CRAIN'S PETROPHYSICAL HANDBOOK | Quick Links |